During the manufacturing process of gears, various errors can occur. Based on their impact on the performance of gear transmission, these errors can be categorized into three groups: errors affecting the accuracy of motion, errors affecting the smoothness of transmission, and errors affecting the uniformity of load distribution. This article provides a brief introduction to the errors that affect the accuracy of motion.
Overview
The requirements for gear transmission in various machines differ depending on their applications, but mainly include the following four aspects:
- Accuracy of motion transmission: This requires that the maximum angular error of the gear within one revolution is limited to a certain range to ensure that the driven component and the driving component move in a coordinated manner.
- Smoothness of transmission: This requires that the instantaneous transmission ratio of the gear transmission does not change significantly. Sudden changes in the instantaneous transmission ratio can cause gear impact, resulting in vibration and noise issues.
- Uniformity of load distribution: This requires that the gear mesh has good tooth surface contact to prevent stress concentration, which can cause localized wear on the tooth surface and affect the gear’s service life.
- Adequate transmission backlash: This requires that there be a certain gap between the non-working meshing surfaces when the gears are meshing. This gap is necessary for storing lubricating oil, compensating for elastic deformation of the gear transmission under load, thermal expansion, and compensating for manufacturing and assembly errors of the gears and other components of the gear mechanism. Otherwise, the gears may seize or sustain damage during transmission.
Previously introduced, the gear processing methods can be divided into profile copying and generation methods based on the principle of tooth profile formation. Taking hobbing as an example, the main factors causing processing errors include the following four aspects:
- Processing errors and installation errors of the hob: These include the radial runout, axial float, and tooth profile angle errors of the hob.
- High-frequency errors in the machine tool transmission chain: During gear processing, it is mainly affected by the indexing chain errors, especially the radial runout and axial float of the indexing worm. In addition, there are also errors in the differential chain.
- Eccentric motion eS: This is caused by processing errors of the machine tool indexing worm and installation eccentricity.
- Geometric eccentricity eG: This is due to the misalignment between the center of the gear tooth ring and the rotational center during gear operation.
When gears are processed using the generation method, the formation of the tooth profile results from the periodic and continuous rolling and cutting of the tool on the gear blank, similar to the meshing transmission process of a rack and pinion pair. Therefore, the processing error is a function of the gear rotation angle and has periodicity, which is a characteristic of gear errors.
Among the four types of errors mentioned above, the errors caused by the first two factors repeat multiple times within one gear rotation and are called short-period errors or high-frequency errors. The errors caused by the latter two factors occur once per gear rotation and are called long-period errors.
In gear accuracy analysis, to facilitate the analysis of the impact of various errors on gear transmission quality, the errors can be divided into axial errors, radial errors, and tangential errors based on their direction relative to the gear.
Based on the impact of various gear errors on the performance of gear transmission, they can be categorized into three groups: errors affecting the accuracy of motion, errors affecting the smoothness of transmission, and errors affecting the uniformity of load distribution. The following introduces the errors affecting the accuracy of motion.
Errors Affecting the Accuracy of Motion
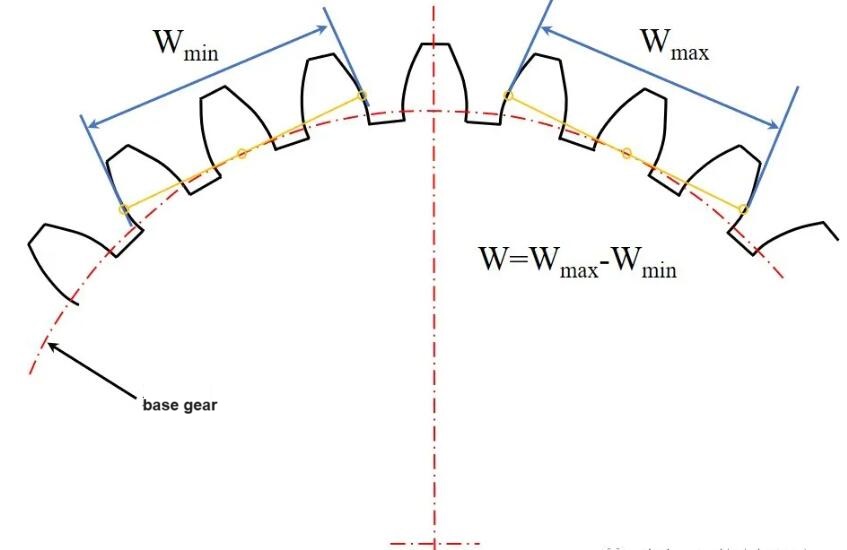
These mainly include five types: the variation in the length of the common normal, the total tangential composite error, the total radial composite error, the radial runout of the gear ring, and the accumulated pitch error.
ΔFw Variation in the Length of the Common Normal (ΔFw)
The variation in the length of the common normal, ΔFw, refers to the difference between the maximum and minimum values of the actual common normal length within one revolution of the gear, as shown in Figure 1.
In hobbing, ΔFw is caused by the motion eccentricity eS, which originates from the eccentricity eT of the machine tool’s indexing worm wheel, as shown in Figure 2.
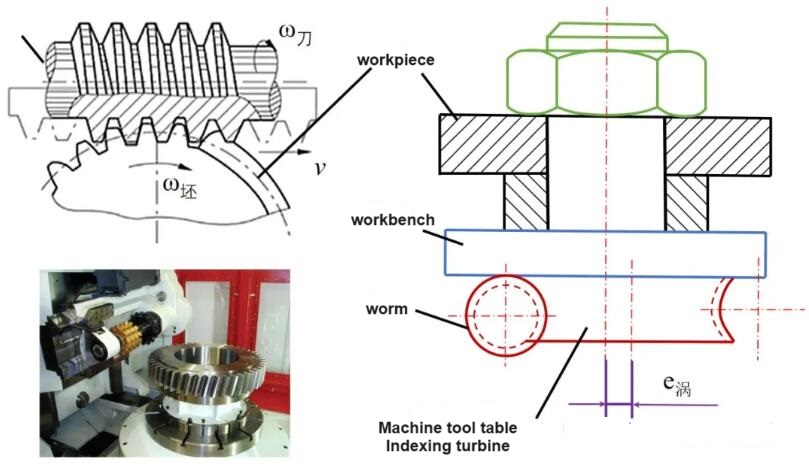
Since the worm wheel is driven by the worm, and assuming the worm’s rotational speed is stable, the linear velocity v at the worm wheel’s meshing point remains constant. Therefore, the angular velocity ωωω is inversely proportional to the rotational radius r (v = ωr). When the indexing worm wheel has an eccentricity eT, it means the rotational radius r is changing, which in turn causes the angular velocity ω to vary. As a result, even if the hob rotates at a constant speed, the rotational speed of the indexing worm wheel and the gear blank it drives will be uneven during the gear cutting process, showing periodic variation. The range of angular velocity variation is from (ω+Δω) to (ω−Δω), with a variation period equal to one rotation of the worktable.
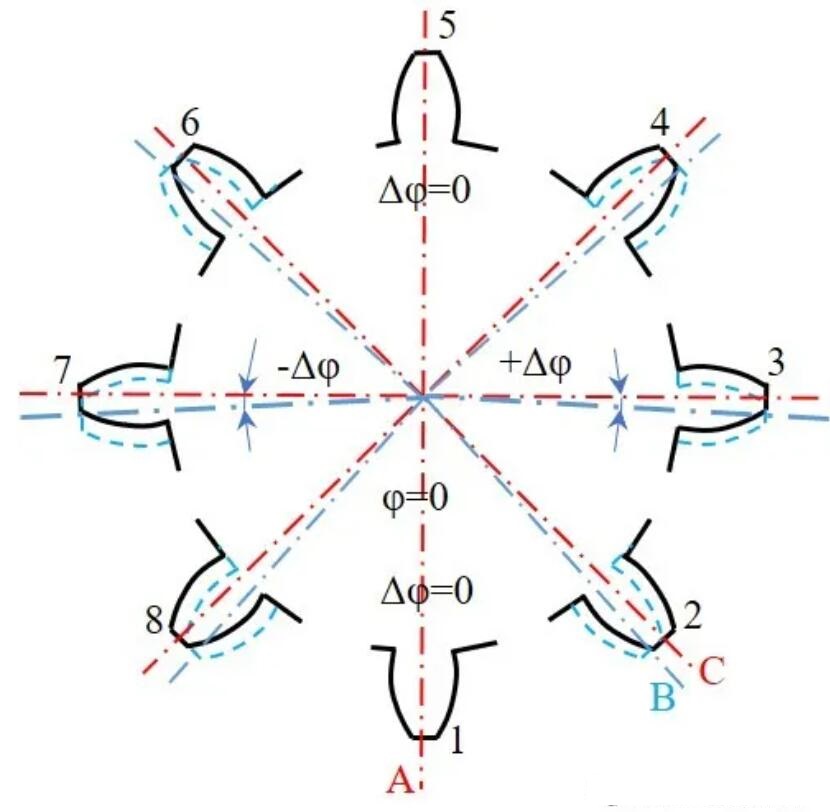
Tangential Composite Error — ΔFi′
This error is measured on a single-face meshing check instrument for gears. The gear under test is mounted on the instrument’s central axis, and while keeping the design center distance aaa unchanged, it is rotated in single-face meshing with the measuring gear to determine the angular error of the gear under test.
The tangential composite error ΔFi′ refers to the maximum difference between the actual and theoretical angles of rotation of the gear under test during one full rotation of the gear when meshing with a precision measuring gear in single-face meshing, calculated in terms of the arc length of the pitch circle, as shown in Figure 4. Alternatively, elements such as racks, worms, and measuring probes may be used instead of the measuring gear.
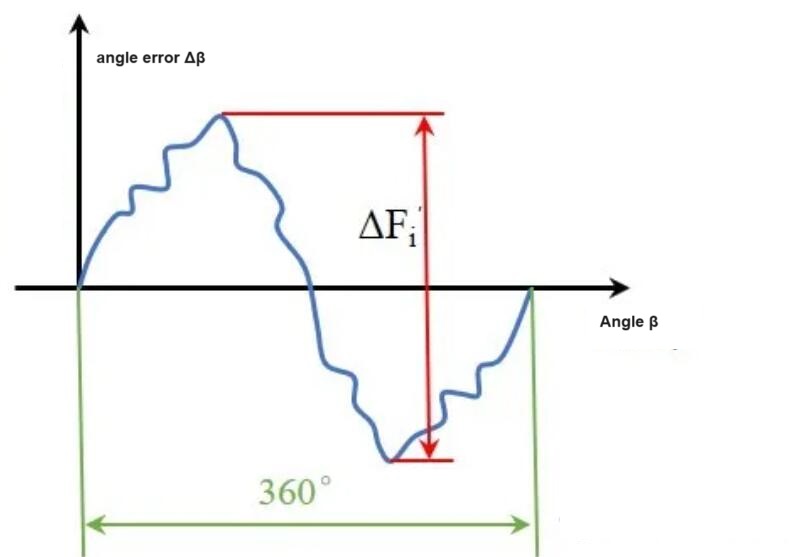
The tangential composite error ΔFi′ reflects the angular rotation error of the gear over one full rotation and is used to indicate the non-uniformity of gear motion. During one full rotation, the rotational speed varies periodically, sometimes speeding up and sometimes slowing down. The tangential composite error ΔFi′ is the result of the combined effects of geometric eccentricity, motion eccentricity, and various short-period errors.
Radial Composite Error — ΔFi”
This error is measured using a double-face meshing check instrument for gears. When the gear under test rotates in double-face meshing with the measuring gear, any radial errors (such as geometric eccentricity) and short-period errors (such as pitch errors, tooth profile errors, etc., which will be discussed later) will cause changes in the double-face meshing center distance.
The radial composite error ΔFi′′ refers to the maximum variation in the double-face meshing center distance during one full rotation of the gear under test when meshing with a precision measuring gear, as shown in Figure 5. The double-face meshing center distance is the center distance between the gear under test and the measuring gear when they are in double-face meshing.
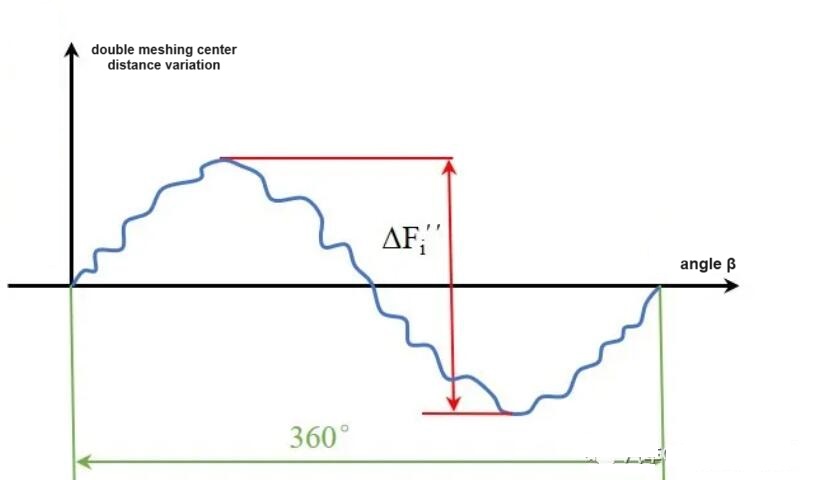
The radial composite error ΔFi′′ mainly reflects radial errors and can substitute for the gear ring radial runout ΔFr. However, due to the higher efficiency of checking the radial composite error ΔFi′′ compared to checking the gear ring radial runout ΔFr, ΔFi′′ is commonly used as the inspection indicator for the first group of gear tolerances (errors affecting the accuracy of motion) in mass production.
Motion eccentricity eS causes tangential errors, leading to uneven distribution of the tooth profiles around the circumference and resulting in accumulated pitch error ΔFp and tooth profile variations. However, motion eccentricity eS does not cause radial errors because, although it causes uneven rotational speed during gear cutting, the distance from the tool to the gear blank center remains constant.
From the above analysis, the following conclusions can be drawn:
- The gear ring radial runout ΔFr and radial composite error ΔFi′′ are primarily caused by geometric eccentricity eG;
- The variation in the length of the common normal ΔFw is caused by motion eccentricity eS;
- The accumulated pitch error ΔFp results from the combined effects of motion eccentricity eS and geometric eccentricity eG;
- The tangential composite error ΔFi′ is the result of the combined influence of both long-period and short-period errors.
Gear Ring Radial Runout — ΔFr
The gear ring radial runout ΔFr refers to the maximum variation of the probe relative to the gear axis, measured during one full rotation of the gear. This measurement is taken in the middle section of the tooth height, where the probe contacts both sides of the tooth slot or tooth surface, as shown in Figure 6.
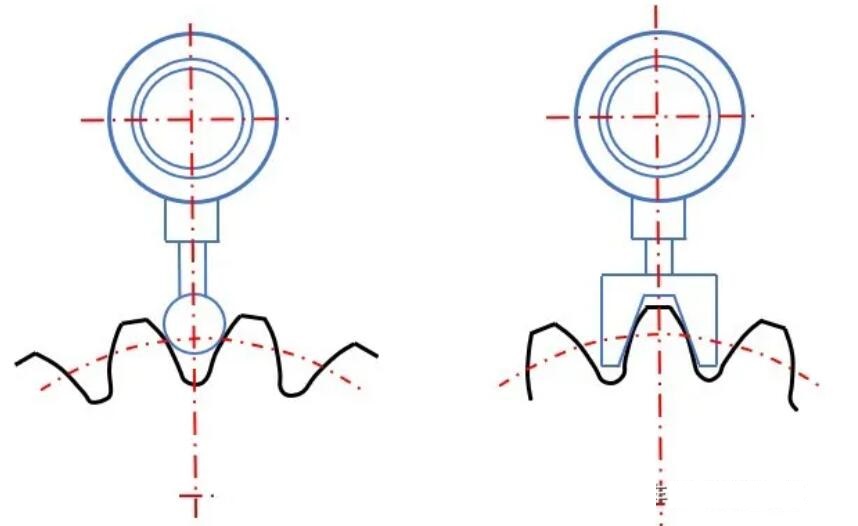
The measurement method for this error is as follows: Using the gear hole as the reference, the probe is sequentially placed into each tooth slot or on each tooth surface. The radial position change of the probe is read from the dial gauge, and the maximum value of this variation is the gear ring radial runout ΔFr.
As mentioned earlier, the gear ring radial runout ΔFr is primarily caused by geometric eccentricity eG. Geometric eccentricity eG may occur during processing, as shown in Figure 7.
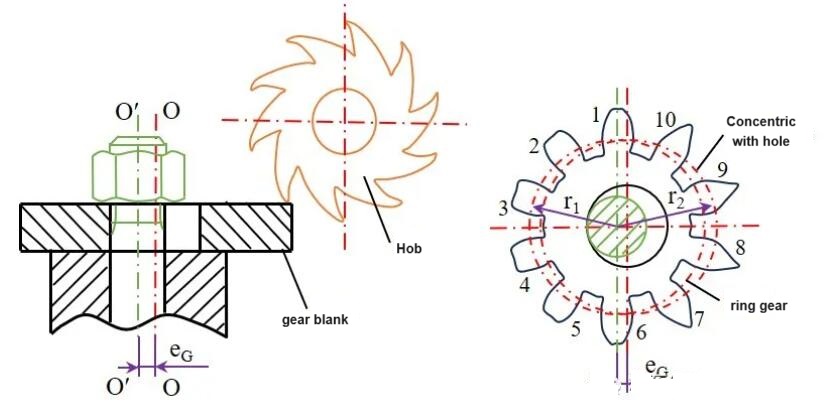
During processing, due to the clearance between the center of the gear blank hole and the central shaft, the center of the hole O does not coincide with the rotational center O′ during gear cutting, resulting in an eccentricity eG.
During the gear cutting process, the distance from the tool to the rotational center O′ remains constant. As a result, the cut gear ring is centered around the rotational center O′, causing the distances from each tooth on the gear ring to the hole center to be unequal and to follow a sinusoidal variation, as shown in Figure 8. This variation has a period of one gear rotation and is a long-period error. Ignoring other errors, the gear ring radial runout ΔFr can be expressed as rmax−rmin=eG.
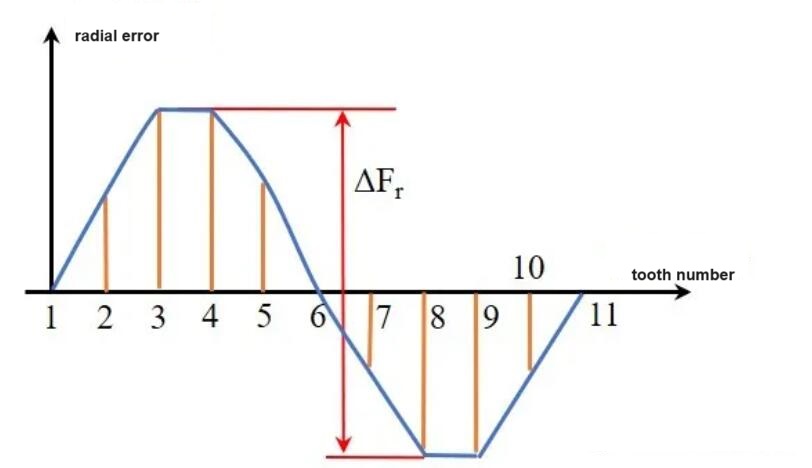
The errors caused by geometric eccentricity eG occur along the radial direction of the gear and are considered radial deviations. When a gear has geometric eccentricity eG, the tooth pitch and tooth thickness along the circle concentric with the hole are uneven. On the side farther from the rotational center O′, the tooth pitch increases and the tooth thickness decreases, while the opposite occurs on the side closer to the rotational center O′, as shown in the right side of Figure 7.
This error varies according to a sinusoidal pattern, causing accumulated pitch errors and changing the backlash in gear transmission. Therefore, geometric eccentricity eG is one of the factors contributing to accumulated pitch errors.
In addition, geometric eccentricity eG can also be introduced during assembly. Assuming no errors in gear processing, if there is a clearance between the hole and the shaft when the gear is mounted on the drive shaft, geometric eccentricity will also occur, with effects similar to the aforementioned errors. Runout of the end face of the gear blank can also introduce additional eccentricity.
Accumulated Pitch Error — ΔFP
The accumulated pitch error ΔFP refers to the maximum difference between the actual and nominal arc lengths between any two teeth on the same side of the pitch circle. It is the algebraic difference between the maximum accumulated pitch deviation ΔFPmax and the minimum accumulated pitch deviation ΔFPmin, as shown in Figure 9.
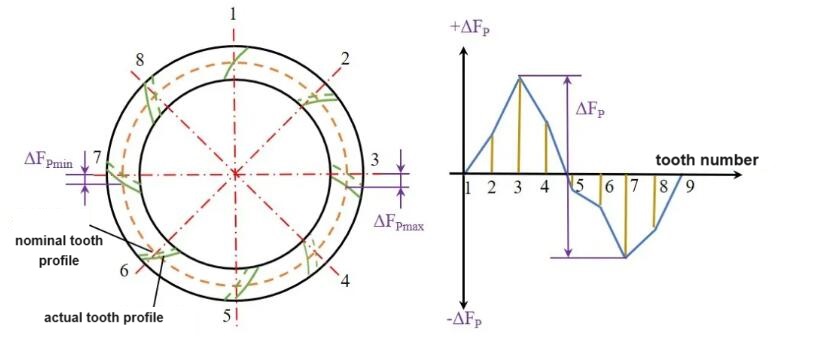
During gear processing, eccentricity inevitably occurs, causing uneven tooth spacing and resulting in accumulated pitch error.
On the production floor, the accumulated pitch error ΔFP is typically measured using relative measurement methods, so this error can also be measured at the middle section of the tooth height.
When necessary, the accumulated pitch error for K pitch circles should also be controlled. The accumulated pitch error ΔFPK refers to the maximum difference between the actual and nominal arc lengths over K pitch circles, where K is an integer from 2 to less than z/2 (where z is the number of teeth), as shown in Figure 9.
The accumulated pitch error also reflects the angular rotation error within one full rotation of the gear. Therefore, accumulated pitch error ΔFP can substitute for tangential composite error ΔFi′ as an indicator of gear motion accuracy. However, there are differences between the two. Accumulated pitch error ΔFP is measured tooth by tooth along the circumference of a circle concentric with the hole, with only one point measured per tooth. The error curve is a broken line, which only indicates the motion error at these discrete points and does not reflect the variation in transmission ratio between points. In contrast, tangential composite error ΔFi′ is measured during continuous single-face meshing operation between the gear under test and the measuring gear. The recorded graph is a continuous curve, reflecting the variation in the gear’s instantaneous transmission ratio, with measurement conditions close to working conditions.
Thank you for reading. Looking forward to serving you with our exceptional gear solutions. #BeyondGears
Read More:


